Foundations Of Quantum Mechanics

• A2.13 B2.21

  Foundations of Quantum Mechanics

  Part II, 2001

  (i) Hermitian operators $\hat{x}, \hat{p}$, satisfy $[\hat{x}, \hat{p}]=i \hbar$. The eigenvectors $|p\rangle$, satisfy $\hat{p}|p\rangle=p|p\rangle$ and $\left\langle p^{\prime} \mid p\right\rangle=\delta\left(p^{\prime}-p\right)$. By differentiating with respect to $b$ verify that

  $$e^{-i b \hat{x} / \hbar} \hat{p} e^{i b \hat{x} / \hbar}=\hat{p}+b$$

  and hence show that

  $$e^{i b \hat{x} / \hbar}|p\rangle=|p+b\rangle$$

  Show that

  $$\langle p|\hat{x}| \psi\rangle=i \hbar \frac{\partial}{\partial p}\langle p \mid \psi\rangle$$

  and

  $$\langle p|\hat{p}| \psi\rangle=p\langle p \mid \psi\rangle .$$

  (ii) A quantum system has Hamiltonian $H=H_{0}+H_{1}$, where $H_{1}$ is a small perturbation. The eigenvalues of $H_{0}$ are $\epsilon_{n}$. Give (without derivation) the formulae for the first order and second order perturbations in the energy level of a non-degenerate state. Suppose that the $r$ th energy level of $H_{0}$ has $j$ degenerate states. Explain how to determine the eigenvalues of $H$ corresponding to these states to first order in $H_{1}$.

  In a particular quantum system an orthonormal basis of states is given by $\left|n_{1}, n_{2}\right\rangle$, where $n_{i}$ are integers. The Hamiltonian is given by

  $$H=\sum_{n_{1}, n_{2}}\left(n_{1}^{2}+n_{2}^{2}\right)\left|n_{1}, n_{2}\right\rangle\left\langle n_{1}, n_{2}\left|+\sum_{n_{1}, n_{2}, n_{1}^{\prime}, n_{2}^{\prime}} \lambda_{\left|n_{1}-n_{1}^{\prime}\right|,\left|n_{2}-n_{2}^{\prime}\right|}\right| n_{1}, n_{2}\right\rangle\left\langle n_{1}^{\prime}, n_{2}^{\prime}\right|,$$

  where $\lambda_{r, s}=\lambda_{s, r}, \lambda_{0,0}=0$ and $\lambda_{r, s}=0$ unless $r$ and $s$ are both even.

  Obtain an expression for the ground state energy to second order in the perturbation, $\lambda_{r, s}$. Find the energy eigenvalues of the first excited state to first order in the perturbation. Determine a matrix (which depends on two independent parameters) whose eigenvalues give the first order energy shift of the second excited state.

  comment

• A3.13 B3.21

  Foundations of Quantum Mechanics

  Part II, 2001

  (i) Write the Hamiltonian for the harmonic oscillator,

  $$H=\frac{p^{2}}{2 m}+\frac{1}{2} m \omega^{2} x^{2}$$

  in terms of creation and annihilation operators, defined by

  $$a^{\dagger}=\left(\frac{m \omega}{2 \hbar}\right)^{\frac{1}{2}}\left(x-i \frac{p}{m \omega}\right), \quad a=\left(\frac{m \omega}{2 \hbar}\right)^{\frac{1}{2}}\left(x+i \frac{p}{m \omega}\right)$$

  Obtain an expression for $\left[a^{\dagger}, a\right]$ by using the usual commutation relation between $p$ and $x$. Deduce the quantized energy levels for this system.

  (ii) Define the number operator, $N$, in terms of creation and annihilation operators, $a^{\dagger}$ and $a$. The normalized eigenvector of $N$ with eigenvalue $n$ is $|n\rangle$. Show that $n \geq 0$.

  Determine $a|n\rangle$ and $a^{\dagger}|n\rangle$ in the basis defined by $\{|n\rangle\}$.

  Show that

  $$a^{\dagger m} a^{m}|n\rangle=\left\{\begin{aligned} \frac{n !}{(n-m) !}|n\rangle, & m \leq n \\ 0, & m>n \end{aligned}\right.$$

  Verify the relation

  $$|0\rangle\langle 0|=\sum_{m=0} \frac{1}{m !}(-1)^{m} a^{\dagger m} a^{m}$$

  by considering the action of both sides of the equation on an arbitrary basis vector.

  comment

• A4.15 B4.22

  Foundations of Quantum Mechanics

  Part II, 2001

  (i) The two states of a spin- $\frac{1}{2}$ particle corresponding to spin pointing along the $z$ axis are denoted by $|\uparrow\rangle$ and $|\downarrow\rangle$. Explain why the states

  $$|\uparrow, \theta\rangle=\cos \frac{\theta}{2}|\uparrow\rangle+\sin \frac{\theta}{2}|\downarrow\rangle, \quad \quad|\downarrow, \theta\rangle=-\sin \frac{\theta}{2}|\uparrow\rangle+\cos \frac{\theta}{2}|\downarrow\rangle$$

  correspond to the spins being aligned along a direction at an angle $\theta$ to the $z$ direction.

  The spin- 0 state of two spin- $\frac{1}{2}$ particles is

  $$|0\rangle=\frac{1}{\sqrt{2}}\left(|\uparrow\rangle_{1}|\downarrow\rangle_{2}-|\downarrow\rangle_{1}|\uparrow\rangle_{2}\right)$$

  Show that this is independent of the direction chosen to define $|\uparrow\rangle_{1,2},|\downarrow\rangle_{1,2}$. If the spin of particle 1 along some direction is measured to be $\frac{1}{2} \hbar$ show that the spin of particle 2 along the same direction is determined, giving its value.

  [The Pauli matrices are given by

  $$\sigma_{1}=\left(\begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array}\right), \quad \sigma_{2}=\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right), \quad \sigma_{3}=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right)$$

  (ii) Starting from the commutation relation for angular momentum in the form

  $$\left[J_{3}, J_{\pm}\right]=\pm \hbar J_{\pm}, \quad\left[J_{+}, J_{-}\right]=2 \hbar J_{3},$$

  obtain the possible values of $j, m$, where $m \hbar$ are the eigenvalues of $J_{3}$ and $j(j+1) \hbar^{2}$ are the eigenvalues of $\mathbf{J}^{2}=\frac{1}{2}\left(J_{+} J_{-}+J_{-} J_{+}\right)+J_{3}^{2}$. Show that the corresponding normalized eigenvectors, $|j, m\rangle$, satisfy

  $$J_{\pm}|j, m\rangle=\hbar((j \mp m)(j \pm m+1))^{1 / 2}|j, m \pm 1\rangle,$$

  and that

  $$\frac{1}{n !} J_{-}^{n}|j, j\rangle=\hbar^{n}\left(\frac{(2 j) !}{n !(2 j-n) !}\right)^{1 / 2}|j, j-n\rangle, \quad n \leq 2 j$$

  The state $|w\rangle$ is defined by

  $$|w\rangle=e^{w J_{-} / \hbar}|j, j\rangle$$

  for any complex $w$. By expanding the exponential show that $\langle w \mid w\rangle=\left(1+|w|^{2}\right)^{2 j}$. Verify that

  $$e^{-w J_{-} / \hbar} J_{3} e^{w J_{-} / \hbar}=J_{3}-w J_{-}$$

  and hence show that

  $$J_{3}|w\rangle=\hbar\left(j-w \frac{\partial}{\partial w}\right)|w\rangle$$

  If $H=\alpha J_{3}$ verify that $\left|e^{i \alpha t}\right\rangle e^{-i j \alpha t}$ is a solution of the time-dependent Schrödinger equation.

  comment

• A2.13 B2.21

  Foundations of Quantum Mechanics

  Part II, 2002

  (i) A Hamiltonian $H_{0}$ has energy eigenvalues $E_{r}$ and corresponding non-degenerate eigenstates $|r\rangle$. Show that under a small change in the Hamiltonian $\delta H$,

  $$\delta|r\rangle=\sum_{s \neq r} \frac{\langle s|\delta H| r\rangle}{E_{r}-E_{s}}|s\rangle,$$

  and derive the related formula for the change in the energy eigenvalue $E_{r}$ to first and second order in $\delta H$.

  (ii) The Hamiltonian for a particle moving in one dimension is $H=H_{0}+\lambda H^{\prime}$, where $H_{0}=p^{2} / 2 m+V(x), H^{\prime}=p / m$ and $\lambda$ is small. Show that

  $$\frac{i}{\hbar}\left[H_{0}, x\right]=H^{\prime}$$

  and hence that

  $$\delta E_{r}=-\lambda^{2} \frac{i}{\hbar}\left\langle r\left|H^{\prime} x\right| r\right\rangle=\lambda^{2} \frac{i}{\hbar}\left\langle r\left|x H^{\prime}\right| r\right\rangle$$

  to second order in $\lambda$.

  Deduce that $\delta E_{r}$ is independent of the particular state $|r\rangle$ and explain why this change in energy is exact to all orders in $\lambda$.

  comment

• A3.13 B3.21

  Foundations of Quantum Mechanics

  Part II, 2002

  (i) Two particles with angular momenta $j_{1}, j_{2}$ and basis states $\left|j_{1} m_{1}\right\rangle,\left|j_{2} m_{2}\right\rangle$ are combined to give total angular momentum $j$ and basis states $|j m\rangle$. State the possible values of $j, m$ and show how a state with $j=m=j_{1}+j_{2}$ can be constructed. Briefly describe, for a general allowed value of $j$, what the Clebsch-Gordan coefficients are.

  (ii) If the angular momenta $j_{1}$ and $j_{2}$ are both 1 show that the combined state $|20\rangle$ is

  

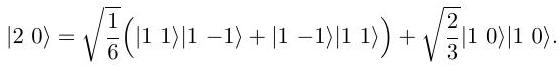
  Determine the corresponding expressions for the combined states $\left|\begin{array}{lll}1 & 0\rangle\end{array}\right\rangle$ and $|0 \quad 0\rangle$, assuming that they are respectively antisymmetric and symmetric under interchange of the two particles.

  If the combined system is in state $|0 \quad 0\rangle$ what is the probability that measurements of the $z$-component of angular momentum for either constituent particle will give the value of 1 ?

  $\left[\right.$ Hint: $\left.\quad J_{\pm}|j m\rangle=\sqrt{(j \mp m)(j \pm m+1)}|j m \pm 1\rangle .\right]$

  comment

• A4.15 B4.22

  Foundations of Quantum Mechanics

  Part II, 2002

  Discuss the consequences of indistinguishability for a quantum mechanical state consisting of two identical, non-interacting particles when the particles have (a) spin zero, (b) spin 1/2.

  The stationary Schrödinger equation for one particle in the potential

  $$-\frac{2 e^{2}}{4 \pi \epsilon_{0} r}$$

  has normalized, spherically symmetric, real wave functions $\psi_{n}(\mathbf{r})$ and energy eigenvalues $E_{n}$ with $E_{0}<E_{1}<E_{2}<\cdots$. What are the consequences of the Pauli exclusion principle for the ground state of the helium atom? Assuming that wavefunctions which are not spherically symmetric can be ignored, what are the states of the first excited energy level of the helium atom?

  [You may assume here that the electrons are non-interacting.]

  Show that, taking into account the interaction between the two electrons, the estimate for the energy of the ground state of the helium atom is

  $$2 E_{0}+\frac{e^{2}}{4 \pi \epsilon_{0}} \int \frac{d^{3} \mathbf{r}_{1} d^{3} \mathbf{r}_{2}}{\left|\mathbf{r}_{1}-\mathbf{r}_{2}\right|} \psi_{0}^{2}\left(\mathbf{r}_{1}\right) \psi_{0}^{2}\left(\mathbf{r}_{2}\right)$$

  comment

• A2.13 B2.21

  Foundations of Quantum Mechanics

  Part II, 2003

  (i) Define the Heisenberg picture of quantum mechanics in relation to the Schrödinger picture. Explain how the two pictures provide equivalent descriptions of observable results.

  Derive the equation of motion for an operator in the Heisenberg picture.

  (ii) For a particle moving in one dimension, the Hamiltonian is

  $$\hat{H}=\frac{\hat{p}^{2}}{2 m}+V(\hat{x}),$$

  where $\hat{x}$ and $\hat{p}$ are the position and momentum operators, and the state vector is $|\Psi\rangle$.

  Eigenstates of $\hat{x}$ and $\hat{p}$ satisfy

  $$\langle x \mid p\rangle=\left(\frac{1}{2 \pi \hbar}\right)^{1 / 2} e^{i p x / \hbar}, \quad\left\langle x \mid x^{\prime}\right\rangle=\delta\left(x-x^{\prime}\right), \quad\left\langle p \mid p^{\prime}\right\rangle=\delta\left(p-p^{\prime}\right) .$$

  Use standard methods in the Dirac formalism to show that

  $$\begin{aligned} &\left\langle x|\hat{p}| x^{\prime}\right\rangle=-i \hbar \frac{\partial}{\partial x} \delta\left(x-x^{\prime}\right) \\ &\left\langle p|\hat{x}| p^{\prime}\right\rangle=i \hbar \frac{\partial}{\partial p} \delta\left(p-p^{\prime}\right) \end{aligned}$$

  Calculate $\left\langle x|\hat{H}| x^{\prime}\right\rangle$ and express $\langle x|\hat{p}| \Psi\rangle,\langle x|\hat{H}| \Psi\rangle$ in terms of the position space wave function $\Psi(x)$.

  Compute the momentum space Hamiltonian for the harmonic oscillator with potential $V(\hat{x})=\frac{1}{2} m \omega^{2} \hat{x}^{2}$.

  comment

• A3.13 B3.21

  Foundations of Quantum Mechanics

  Part II, 2003

  (i) What are the commutation relations satisfied by the components of an angular momentum vector $\mathbf{J}$ ? State the possible eigenvalues of the component $J_{3}$ when $\mathbf{J}^{2}$ has eigenvalue $j(j+1) \hbar^{2}$.

  Describe how the Pauli matrices

  $$\sigma_{1}=\left(\begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array}\right), \quad \sigma_{2}=\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right), \quad \sigma_{3}=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right)$$

  are used to construct the components of the angular momentum vector $\mathbf{S}$ for a spin $\frac{1}{2}$ system. Show that they obey the required commutation relations.

  Show that $S_{1}, S_{2}$ and $S_{3}$ each have eigenvalues $\pm \frac{1}{2} \hbar$. Verify that $\mathbf{S}^{2}$ has eigenvalue $\frac{3}{4} \hbar^{2} .$

  (ii) Let $\mathbf{J}$ and $|j m\rangle$ denote the standard operators and state vectors of angular momentum theory. Assume units where $\hbar=1$. Consider the operator

  $$U(\theta)=e^{-i \theta J_{2}}$$

  Show that

  $$\begin{aligned} &U(\theta) J_{1} U(\theta)^{-1}=\cos \theta J_{1}-\sin \theta J_{3} \\ &U(\theta) J_{3} U(\theta)^{-1}=\sin \theta J_{1}+\cos \theta J_{3} \end{aligned}$$

  Show that the state vectors $U\left(\frac{\pi}{2}\right)|j m\rangle$ are eigenvectors of $J_{1}$. Suppose that $J_{1}$ is measured for a system in the state $|j m\rangle$; show that the probability that the result is $m^{\prime}$ equals

  $$\left|\left\langle j m^{\prime}\left|e^{i \frac{\pi}{2} J_{2}}\right| j m\right\rangle\right|^{2}$$

  Consider the case $j=m=\frac{1}{2}$. Evaluate the probability that the measurement of $J_{1}$ will result in $m^{\prime}=-\frac{1}{2}$.

  comment

• A4.15 B4.22

  Foundations of Quantum Mechanics

  Part II, 2003

  Discuss the quantum mechanics of the one-dimensional harmonic oscillator using creation and annihilation operators, showing how the energy levels are calculated.

  A quantum mechanical system consists of two interacting harmonic oscillators and has the Hamiltonian

  $$H=\frac{1}{2} \hat{p}_{1}^{2}+\frac{1}{2} \hat{x}_{1}^{2}+\frac{1}{2} \hat{p}_{2}^{2}+\frac{1}{2} \hat{x}_{2}^{2}+\lambda \hat{x}_{1} \hat{x}_{2}$$

  For $\lambda=0$, what are the degeneracies of the three lowest energy levels? For $\lambda \neq 0$ compute, to lowest non-trivial order in perturbation theory, the energies of the ground state and first excited state.

  [Standard results for perturbation theory may be stated without proof.]

  comment

• A2.13 B2.22

  Foundations of Quantum Mechanics

  Part II, 2004

  (i) The creation and annihilation operators for a harmonic oscillator of angular frequency $\omega$ satisfy the commutation relation $\left[a, a^{\dagger}\right]=1$. Write down an expression for the Hamiltonian $H$ in terms of $a$ and $a^{\dagger}$.

  There exists a unique ground state $|0\rangle$ of $H$ such that $a|0\rangle=0$. Explain how the space of eigenstates $|n\rangle, n=0,1,2, \ldots$ of $H$ is formed, and deduce the eigenenergies for these states. Show that

  $$a|n\rangle=\sqrt{n}|n-1\rangle, \quad a^{\dagger}|n\rangle=\sqrt{n+1}|n+1\rangle$$

  (ii) Write down the number operator $N$ of the harmonic oscillator in terms of $a$ and $a^{\dagger}$. Show that

  $$N|n\rangle=n|n\rangle$$

  The operator $K_{r}$ is defined to be

  $$K_{r}=\frac{a^{\dagger r} a^{r}}{r !}, \quad r=0,1,2, \ldots$$

  Show that $K_{r}$ commutes with $N$. Show also that

  $$K_{r}|n\rangle= \begin{cases}\frac{n !}{(n-r) ! r !}|n\rangle & r \leq n \\ 0 & r>n\end{cases}$$

  By considering the action of $K_{r}$ on the state $|n\rangle$ show that

  $$\sum_{r=0}^{\infty}(-1)^{r} K_{r}=|0\rangle\langle 0|$$

  comment

• A3.13 B3.21

  Foundations of Quantum Mechanics

  Part II, 2004

  (i) A quantum mechanical system consists of two identical non-interacting particles with associated single-particle wave functions $\psi_{i}(x)$ and energies $E_{i}, i=1,2, \ldots$, where $E_{1}<E_{2}<\ldots$ Show how the states for the two lowest energy levels of the system are constructed and discuss their degeneracy when the particles have (a) spin 0 , (b) spin $1 / 2$.

  (ii) The Pauli matrices are defined to be

  $$\sigma_{1}=\left(\begin{array}{cc} 0 & 1 \\ 1 & 0 \end{array}\right), \quad \sigma_{2}=\left(\begin{array}{cc} 0 & -i \\ i & 0 \end{array}\right), \quad \sigma_{3}=\left(\begin{array}{cc} 1 & 0 \\ 0 & -1 \end{array}\right)$$

  State how the spin operators $s_{1}, s_{2}, s_{3}$ may be expressed in terms of the Pauli matrices, and show that they describe states with total angular momentum $\frac{1}{2} \hbar$.

  An electron is at rest in the presence of a magnetic field $\mathbf{B}=(B, 0,0)$, and experiences an interaction potential $-\mu \boldsymbol{\sigma} \cdot \mathbf{B}$. At $t=0$ the state of the electron is the eigenstate of $s_{3}$ with eigenvalue $\frac{1}{2} \hbar$. Calculate the probability that at later time $t$ the electron will be measured to be in the eigenstate of $s_{3}$ with eigenvalue $\frac{1}{2} \hbar$.

  Part II 2004

  comment

• A4.15 B4.22

  Foundations of Quantum Mechanics

  Part II, 2004

  The states of the hydrogen atom are denoted by $|n l m\rangle$ with $l<n,-l \leq m \leq l$ and associated energy eigenvalue $E_{n}$, where

  $$E_{n}=-\frac{e^{2}}{8 \pi \epsilon_{0} a_{0} n^{2}} .$$

  A hydrogen atom is placed in a weak electric field with interaction Hamiltonian

  $$H_{1}=-e \mathcal{E} z$$

  a) Derive the necessary perturbation theory to show that to $O\left(\mathcal{E}^{2}\right)$ the change in the energy associated with the state $|100\rangle$ is given by

  $$\Delta E_{1}=e^{2} \mathcal{E}^{2} \sum_{n=2}^{\infty} \sum_{l=0}^{n-1} \sum_{m=-l}^{l} \frac{|\langle 100|z| n l m\rangle|^{2}}{E_{1}-E_{n}}$$

  The wavefunction of the ground state $|100\rangle$ is

  $$\psi_{n=1}(\mathbf{r})=\frac{1}{\left(\pi a_{0}^{3}\right)^{1 / 2}} e^{-r / a_{0}}$$

  By replacing $E_{n}, \forall n>1$, in the denominator of $(*)$ by $E_{2}$ show that

  $$\left|\Delta E_{1}\right|<\frac{32 \pi}{3} \epsilon_{0} \mathcal{E}^{2} a_{0}^{3}$$

  b) Find a matrix whose eigenvalues are the perturbed energies to $O(\mathcal{E})$ for the states $|200\rangle$ and $|210\rangle$. Hence, determine these perturbed energies to $O(\mathcal{E})$ in terms of the matrix elements of $z$ between these states.

  [Hint:

  $$\begin{aligned} \langle n l m|z| n l m\rangle &=0 & & \forall n, l, m \\ \left\langle n l m|z| n l^{\prime} m^{\prime}\right\rangle &=0 & & \forall n, l, l^{\prime}, m, m^{\prime}, \quad m \neq m^{\prime} \end{aligned}$$

  comment